Contents
Full Beam reinforcement manual calculation as per IS 456-2000 code | Beam design | structural design |
In this article I will explain you complete manual design of Ground floor beam reinforcement details as per the IS 456-2000 code provisions.
In my previous blog the complete calculation of dead load is explained please click here to read article.
Basically beam is the horizontal member in building structure which is exists in X direction and Y direction condition as per the architectural plan. The design of beam reinforcement depends upon the loading condition. If the total load on the building is less than less reinforcement bars are required to build the structure.
For the present case ground floor building is considered which is consisting of three cases of beams namely B1, B2 and B3.
The typical beam layout is shown in the below figure for the beam reinforcement calculation.
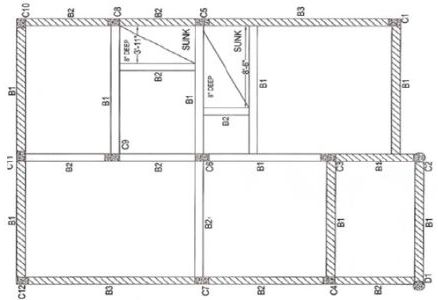
The above figure shows the beam layout of ground floor building which is consisting of three case of beams namely B1, B2 and B3 as per the architectural floor plan. For the beams dimensions of beam is taken as 300mmX400mm cross section, 250mmX350mm, and 300mmX350mm
The load acting on the beam through the gravity load for beam 1 is 19.696kN/m, for beam 1 is 10.55kN/m, and finally for Beam 3 it is considered as 9.584kN/m.
Beam 1 design details as per IS 456-2000 code
Calculation of load
a) UDL = 19.696 kN/m
b) Self weight of beam = γc× b ×D
Where γc is unit weight of concrete
For plain concrete γc is 24kN/m3 and for reinforced concrete γc is 25kN/m3
= 25×0.3×0.45
= 3.225 kN/m
Total load = sum of UDL and self weight of beam
= 19.696 + 3.22
= 22.92 kN/m
Factored load = γp×load
Where γp is the factor of safety as per IS 456 it is considered as 1.5
= 1.5 ×22.92
= 34.3815 kN/m
Calculation of moment and moment of resistance :
Mmax = (wxlxl)/8
= (34.3815X4.8X4.8)/8 (where l = span of the beam =4.8m)
=99.0 KN-M
and
MOR = 0.138× fckXbXdXd (Fe415 Xu= 0.48d)
= 0.138×20×300×400X400
= 132.48KN-M
- Since (MOR) ˃ Mmax , so beam is designed as singly reinforced
Reinforcement:
As a is designed for under -reinforcement case.
i.e˂ Xu<Xumax
Then from IS 456, pg 96 ,Annex 9.
Mmax= 0.87XfyXAstXd (1-(fyXAst/fckXbXd))
1000000×99 = 0.87×415×Ast×400(1-(415XAst/20X300X400))
Ast= 773.57 mm2
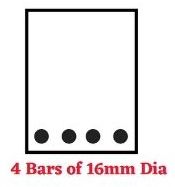
By assuming 16mm diameter bars
Max provide bar of dia =16mm
Area of individual bars =(ΠXdXd)/4
=(ΠX16X16)/4
= 201.06 mm2
Number of bars required = (total steel/area of individual bars)
= 3.84 4 no’s
Approximately 4 bars of 16mm diameter required
Ast provided =((ΠX16X16)/4)X4
= 804.24mm2
- Check for reinforcement: ( IS 456 ,pg .46 ,47 – cl- 20.5)
- min reinforcement
Ast min =(0.85XbXd)/fy (b=300, d=400)
=(0.85X300X400)/fy
= 245.78mm2
Ast min˂ Ast provided = 804.24mm2
2. max reinforcement = 4% of gross area of bars
= 0.04×b×D
= 0.04×300×400
= 4800mm2 ˃ (Ast) provided.
Beam 2 reinforcement details as per IS 456-2000 code
Calculation of load
a) UDL = 10.55 kN/m
b) Self weight of beam = γc× b ×D
Where γc is unit weight of concrete
For plain concrete γc is 24kN/m3 and for reinforced concrete γc is 25kN/m3
self weight of beam = 25×0.25×0.38
= 2.375
Total load = 10.55+ 2.375
=12.92kN/m
Factored load = γp×load
Where γp is the factor of safety as per IS 456 it is considered as 1.5
=1.5X12.92
= 19.38 kN/m
Calculation of moment and moment of resistance :
Mmax = (wxlxl)/8
=(19.38X4.4X4.4)/8 (where l = span of the beam =4.4m)
=46.949 KN-M
and
MOR = 0.138× fckXbXdXd (Fe415 Xu= 0.48d)
= 0.138×20×250×350X350
= 84.52KN-M
- Since (MOR) ˃ Mmax so beam is designed as singly reinforced
Reinforcement :
As a is designed for under -reinforcement case.
i.e˂ Xu<Xumax
Then from IS 456, pg 96 ,Annex 9.
Mmax= 0.87XfyXAstXd (1-(fyXAst/fckXbXd))
1000000×46.949 = 0.87×415×Ast×380(1-(415XAst/20X250X380))
Ast= 412.22mm2
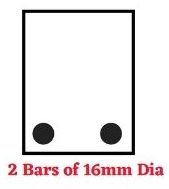
By assuming 16mm diameter bars
Max provide bar of dia =16mm
Area of individual bars =(ΠXdXd)/4
=(ΠX16X16)/4
= 201.06 mm2
Number of bars required = (total steel/area of individual bars)
= 2 no’s
Approximately 2 bars of 16mm diameter required
Ast provided =((ΠX16X16)/4)X2
= 402.12mm2
- Check for reinforcement: ( IS 456 ,pg .46 ,47 – cl- 20.5)
- min reinforcement
Ast min =(0.85XbXd)/fy (b=300, d=400)
=(0.85X250X380)/fy
= 194.58mm2
Ast min˂ Ast provided = 402.12mm2
2. max reinforcement = 4% of gross area of bars
= 0.04×b×D
= 0.04×250×380
= 3800mm2 ˃ (Ast) provided.
Beam 3 reinforcement details as per IS 456-2000 code
Calculation of load
a) UDL = 9.584 kN/m
b) Self weight of beam = γc× b ×D
Where γc is unit weight of concrete
For plain concrete γc is 24kN/m3 and for reinforced concrete γc is 25kN/m3
self weight of beam = 25×0.3×0.38
= 2.85kN/m
Total load = 9.584+ 2.85
=12.434kN/m
Factored load = γp×load
Where γp is the factor of safety as per IS 456 it is considered as 1.5
=1.5X12.434
= 18.651 kN/m
Calculation of moment and moment of resistance:
Mmax = (wxlxl)/8
=(18.651X3.94X3.94)/8 (where l = span of the beam =4.4m)
=36.19 KN-M
and
MOR = 0.138× fckXbXdXd (Fe415 Xu= 0.48d)
= 0.138×20×300×350X350
= 101.43 KN-M
- Since (MOR) ˃ Mmax so beam is designed as singly reinforced
Reinforcement :
As a is designed for under -reinforcement case.
i.e˂ Xu<Xumax
Then from IS 456, pg 96 ,Annex 9.
Mmax= 0.87XfyXAstXd (1-(fyXAst/fckXbXd))
1000000×36.19 = 0.87×415×Ast×350(1-(415XAst/20X300X350))
Ast= 304.67 mm2
By assuming 16mm diameter bars
Max provide bar of dia =16mm
Area of individual bars =(ΠXdXd)/4
=(ΠX16X16)/4
= 201.06 mm2
Number of bars required = (total steel/area of individual bars)
= 2 no’s
Approximately 2 bars of 16mm diameter required
Ast provided =((ΠX16X16)/4)X2
= 402.12mm2

- Check for reinforcement: ( IS 456 ,pg .46 ,47 – cl- 20.5)
- min reinforcement
Ast min =(0.85XbXd)/fy (b=300, d=350)
=(0.85X300X350)/fy
= 215.06mm2
Ast min˂ Ast provided = 402.12mm2
2. max reinforcement = 4% of gross area of bars
= 0.04×b×D
= 0.04×300×350
= 4200mm2 ˃ (Ast) provided.
Final Beam reinforcement details for B1, B2 and B3
|
S. No |
Beam ID |
Ast |
Reinforcement type |
Number of bars required |
|
1 |
B1 |
773.57 |
Single reinforced |
4 |
|
2 |
B2 |
402.22 |
Single reinforced |
2 |
| 3 | B3 |
304.67 |
Single reinforced |
2 |
The complete concepts of beam reinforcement details is explained in my YouTube Channel Civil Engineering by shravan please click here to read.
Conclusions for beam reinforcement details
Well now the above explained concepts are related to the complete beam reinforcement details calculations for ground floor building structure as per IS 456-2000 code.
The reinforcement details are calculated for three types of beams namely B1, B2, and B3 sections. For B1 it is required 4 bars of 16mm diameter, for B2 and B3 it is required 2 bars of 16mm diameter at bottom.
Please follow our previous posts here
Cost estimation of marbles and tiles for 1000sft and1500sft slab area click here to read
Volume of concrete per cubic meter click here to read
What is slab? Types of slabs? Difference between one way slab and two way slab ? click here to read
What is minimum and maximum center to center distance between RCC columns click here to read
What is cost estimation of 3BHK building click here to read
Please watch interesting concepts in my YouTube channel Civil engineering by shravan. Please feel free to text us at contact us page for any quarries.
Thank You
Your Shravan
Have a good day.